Neurophysiology Modules

Module 2: Membrane potentials

---

Ion Concentrations Across Membranes Generate Membrane Potentials

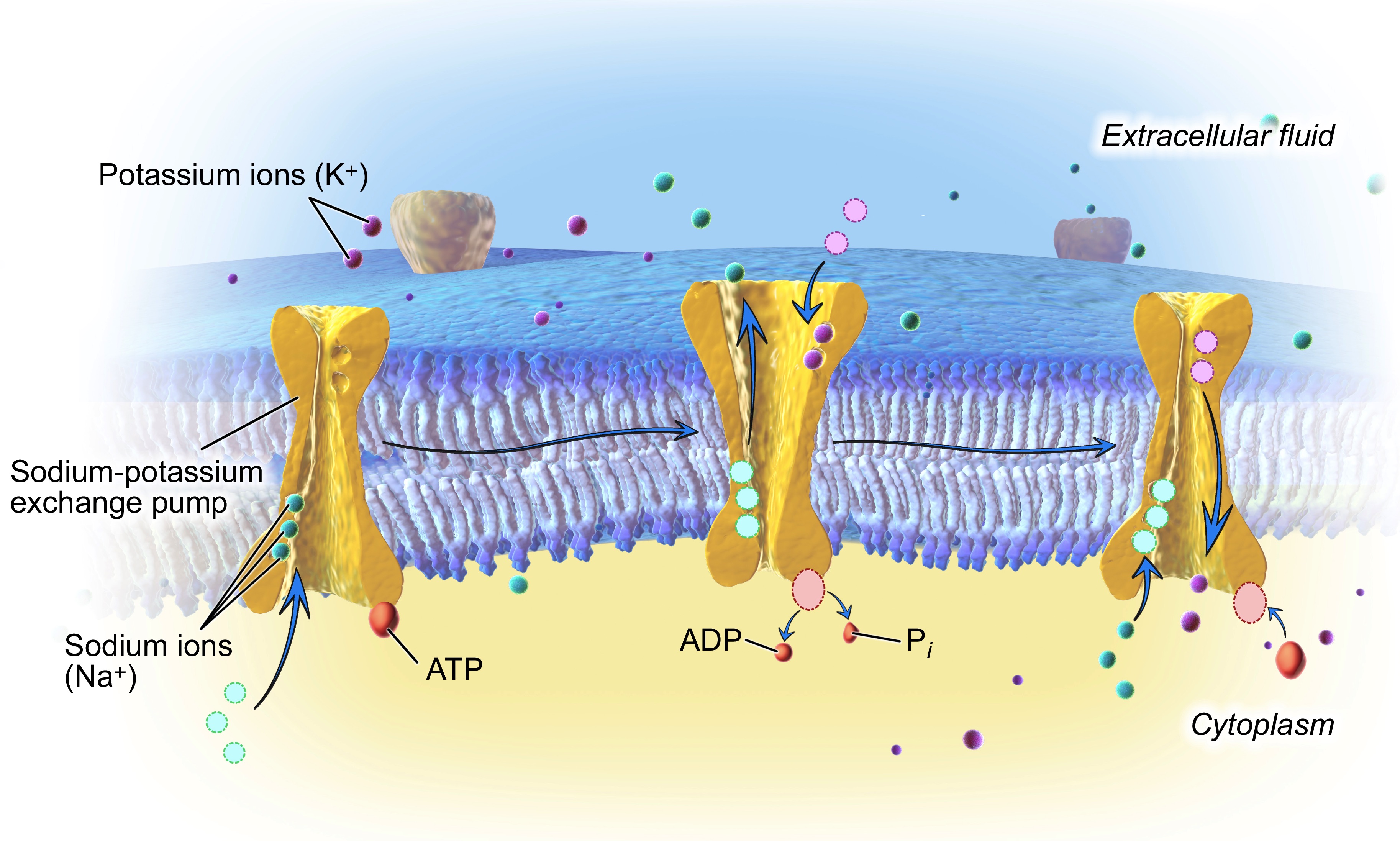
The lipid bilayer that forms the wall of a neuron (or glial cell) is not permeable to charged ions.  However, there are a number of types of ion channels that allow ions to move down their concentration gradient across the membrane, and ion transporters that allow ions to move against their concentration gradient (from low concentration to high concentration).  One of the best examples of an ion transporter is the sodium-potassium pump (also called Na+/K+ pump or Na+/K+ ATPase), which pumps potassium into a cell and sodium out of a cell, both against their concentration gradients, with the use of ATP to provide energy for the process.   The actions of this pump sequester K+ inside of neurons and extrude Na+ from neurons (in other words, the sodium potassium pump causes intracellular K+ to be high and intracellular Na+ to be low, relative to the extracellular fluid).

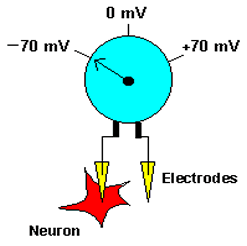The term membrane potential  refers to the electrical potential difference across the membrane of a cell.  To accurately measure the membrane potential, an investigator would need to place one electrode inside the cell and another outside, and compare the voltage on the two sides (see figure to left).  The membrane potential is generated by the number of charged particles (positive and negative) on the two sides of the membrane.  Most of these charged particles are ions, although some proteins inside cells are negatively charged, and contribute to producing the membrane potential.  Virtually all cells have an interior that is negatively charged with respect to the outside.

Equilibrium Potentials

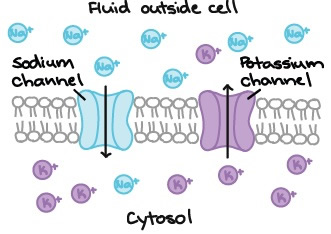If ions were uncharged, then we would only need to worry about the concentration gradient to understand ion movements across a membrane.  However, since ions are charged, they are attracted to ions with opposite electric charges (positive to negative, and vice versa), and repelled by ions of like charges.  Positively charged ions are called cations, and negatively charged ions are called anions. As an example, let’s consider a membrane that is freely permeable to Na+ and K+ ions, but there are no other cations and no anions present.  Since both ions have the same charge, then concentration gradient is the only factor that affects their movement.

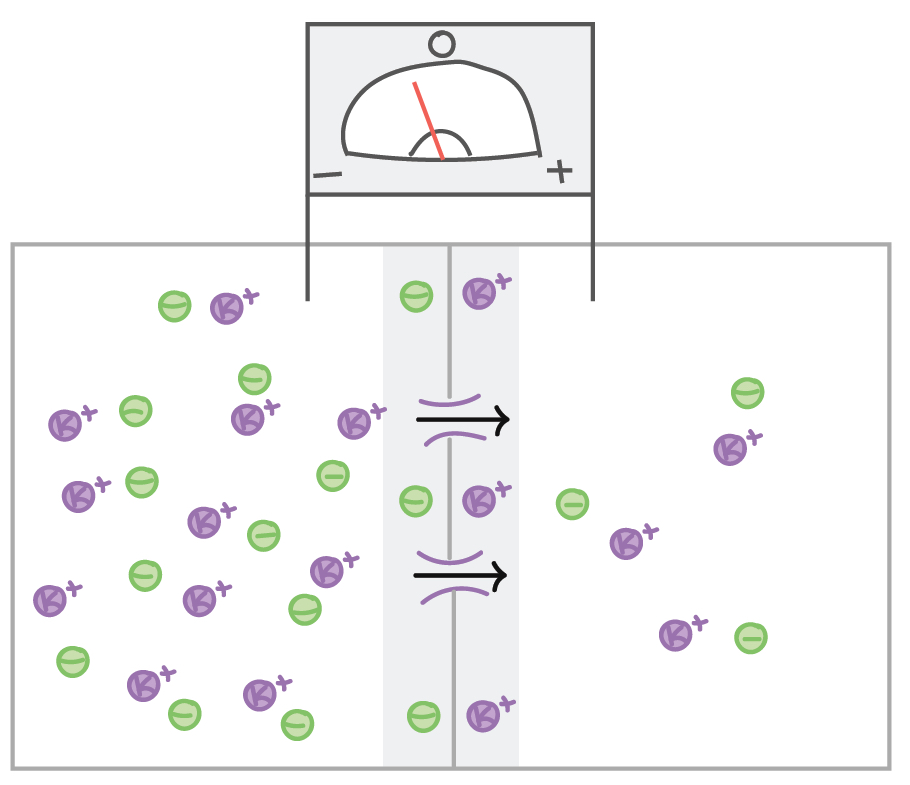Let’s next consider a cell that has many K+ ions inside and few outside (which is the normal case for neurons, due to the activity of the sodium-potassium pump), but the resting membrane potential is 0 due to the presence of negative ions balancing the positive ones. If channels that allow K+ to pass are opened, K+ will move down its concentration gradient (from inside to outside the cell).  As a result, there will be a loss of positive charge from the inside of the cell, so the inside will become negatively charged with respect to the outside.

However, if an investigator placed an electrode outside of the cell and delivered a positive charge, the flow of K+ from inside to outside the cell would be opposed, as the K+ ions would be repelled by the positive charge that is being delivered.  The charge that is needed to keep K+ from diffusing from high concentration to low concentration when the K+ channels open is called the equilibrium potential.  The equilibrium potential is sometimes called a reversal potential, as it is the charge that is needed to prevent ions from moving down their concentration gradient.

As you might imagine, the exact charge that is needed to prevent the movement of ions down a concentration gradient can be calculated mathematically, with the use of the Nernst equation.    However, we will not use such mathematical approaches in this course.

One take-away from the Nernst equation is relevant to us: the charge that needs to be delivered to prevent the movement of ions is dependent on the difference in concentration of the ions across the membrane.   If there is a large concentration difference, then a larger charge has to be delivered to oppose ionic movement than when the concentration gradient is small. 

Let’s apply these principles to the K+ distribution across a membrane.  With normal inside and outside concentrations of K+, a charge of -88 mV has to be present (inside relative to outside, or 88 mV more positive charge outside than inside) to keep K+ from changing concentration across the membrane.  This makes sense, as K+ is a positive ion, and is attracted by a negative charge.    If the charge inside the neuron (relative to the outside) was made extremely negative (let’s say —100 mV), then K+ would move against its concentration gradient and enter the cell.

I Don’t Get it—Why is the Inside of a Neuron Negatively Charged?

From this discussion, it may be empirically difficult to visualize why the interior of a neuron is negatively charged.  There are three reasons: The sodium-potassium pump removes three intracellular Na+ ions for every two K+ ions it lets in.    Proteins that carry a negative charge are in high concentration inside a cell, and in low concentration outside. Neuronal membranes tend to be leakier for K+ than for Na+.  This causes a net loss of K+ cations from the inside of the neuron, making it more negative. This is the major factor.

The Membrane Potential of Neurons is Dependent on Several Ions

Most neurons have channels that allow the passage of Na+, K+, and Cl-. Thus, all of these ions contribute to the membrane potential of neurons.

As noted above, the sodium-potassium pump causes the intracellular K+ levels and the extracellular Na+ levels to be high, relative to the other side of the membrane. Since Na+ is high outside the neuron, the concentration gradient favors the entry of Na+ into the cell. The equilibrium potential for Na+ is about 60 mV, as a positive internal charge would oppose the entry of Na+ when Na+ channels open (since cations are repelled by a positive charge).

The membrane potential for a resting neuron is between the equilibrium potentials for K+ and Na+, usually about —70 mV. This is much closer to the equilibrium potential for K+ than that of Na+. WHY? The main reason is that the resting neuronal membrane is leakier for K+ than for Na+.

Why doesn’t Cl- contribute much to the resting membrane potential? The main reason is that most neurons don’t actively transport Cl-, so concentration gradients are the main factor that govern the movement of the ion. The equilibrium potential for Cl- is also near the resting membrane potential of a neuron. Generally, Cl- is higher outside the neuron than inside, as the negative intracellular potential repels the anion.

 

---